views
Analyzing the Rate Equation

Identify the rate equation from the reaction. The rate equation can help you determine the order of the reaction. This equation shows the increase or decrease of a particular substance with respect to time. Other equations related to the chemical reaction will not help you identify the order of the reaction.
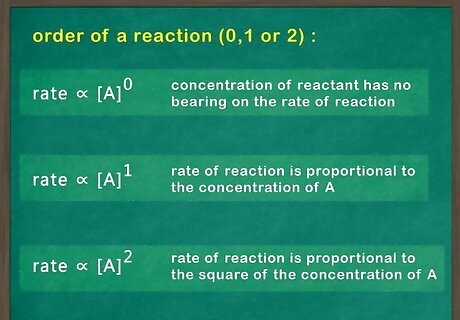
Identify the order of each reactant. Each reactant listed in the rate equation will have an exponent of either 0, 1, or 2 (above 2 is very rare). That exponent denotes the order of that reactant. Looking at each exponent: A zero means that the concentration for that reactant has no bearing on the rate of reaction. A one means that increasing the concentration of this reactant will increase the rate of the reaction in a linear way (doubling the reactant doubles the rate). A two means that the rate of the reaction will increase by the square of the increased concentration (doubling the reactant will increase the rate by four times). Zero order reactants are often not listed in the rate equation, since any number to the zeroth power is equal to one.

Add the order for all reactants together. The overall order of a reaction is the sum of each reactants' orders. Add the exponents of each reactant to find the overall reaction order. This number is usually less than or equal to two. For example, if reactant one is first order (an exponent of 1) and reactant two is first order (an exponent of 1) then the overall reaction would be a second order reaction.
Graphing Data Points
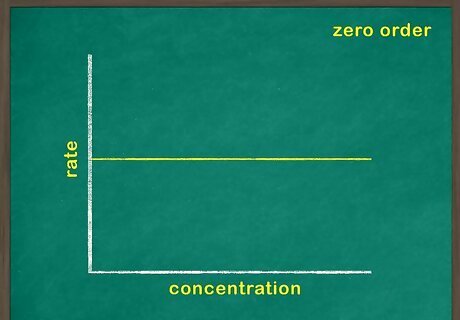
Find the variables that create a linear graph of the reaction. A linear graph refers to a graph that has a constant rate of change. In other words, the dependent variable will change as much in the first second as it does the second, third, and so on. A linear graph looks like a straight line on the page.
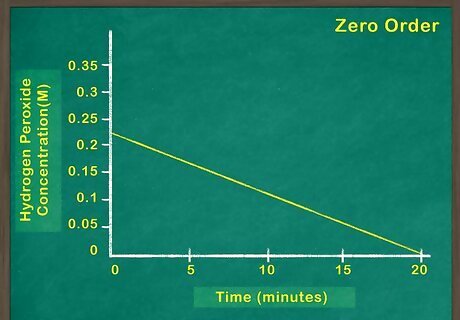
Graph concentration of reactant versus time. This will indicate how much reactant remains at any given point during the reaction. If this graph is linear, it means that the concentration of the reactant has no bearing on the rate at which the reaction proceeds. In this case, the reactant is a zeroth order reactant.
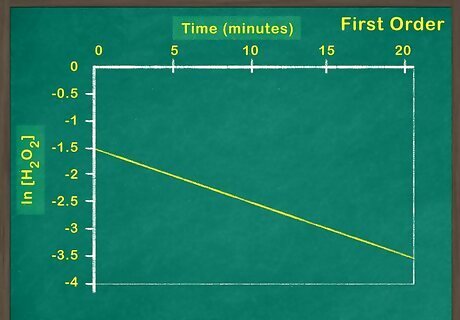
Plot the natural log of the concentration of reactant versus time. If graphing the natural log of the reactant results in a linear graph, the reactant is a first order reactant. This means that the concentration of the reactant impacts the rate of reaction. If the graph is not linear, you must graph test for a second order reaction.
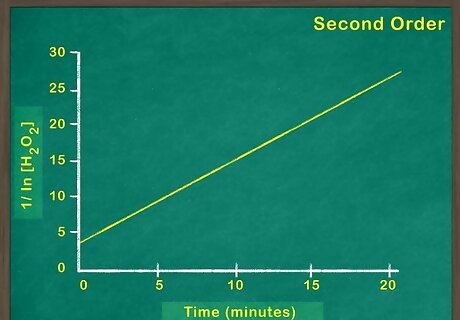
Make a graph of [1/concentration of reactant] versus time. A linear graph of [1/concentration of reactant] indicates a second order reaction. This means that the rate of the reaction will increase by the square of any increase in reactant. If this graph is not linear, you must try graphing zeroth and first order reactions.

Find the sum of the order for all reactants. Once you have found a linear graph for each reactant, you will know the order of each reactant. This allows you to calculate the overall reaction order. Add all reactant orders together. This is your reaction order for the whole reaction.
Solving Practice Problems
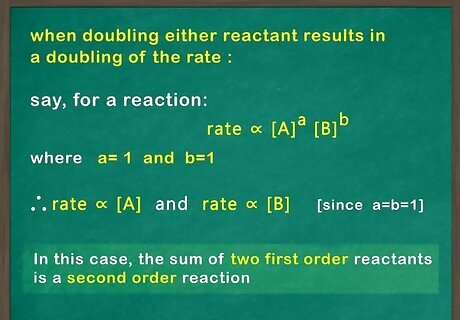
Determine the order of a reaction when doubling either reactant results in a doubling of the rate. You must know that if doubling the concentration of a reactant causes the rate to double, then that reactant is of the first order. In this case, both reactants are first order. The sum of two first order reactants is a second order reaction.

Find the order of a reaction in which doubling both reactants leads to no change in rate. If changing the concentration of a reactant produces no change in rate, that reactant is said to be of the zeroth order. In this case, both reactants are of the zeroth order. Reacting two zeroth order reactions gives you an overall reaction order of zero.

Determine the order of a reaction in which doubling one reactant quadruples the rate. The reactant that impacts the reaction rate is of the second order. The second reactant has no impact on rate and is of the zeroth order. The sum of orders is two, thus this is a second order reaction.



















Comments
0 comment